Economic Principles: How the Market Works

Chapter 6: Production Choice in the Short Run

• Short run: some factors are given
• Why must we maximize profits?
• The constraints: capital market, labour market, good market
• Technology with decreasing return
• Cost curves: decreasing returns
• Profit maximization

  •  a first graphical approach
  •  using algebra
  •  using the marginal cost curves
  

To be remembered:

•  profit (definition)

•  marginal cost

•  average cost

•  decreasing returns

•  profitability threshold

Be aware of schizophrenia

• Listening to many commentators of daily news, it looks like if most citizens were victims of schizophrenia 1! Those commentators always oppose the gentle and kind behaviour of (the poor) consumers to the brutal and selfish behaviour of (the rich) producers. But aren't consumers and producers the same persons? Aren't we each one of us both producer and consumer? So to have such illogical pattern of behaviour we must surely be schizophrenic.
• But don't worry. The truth is that the behaviour of the producer and the behaviour of the consumer follow the same logic; the kind of logic that we studied in detail in the previous chapters. In fact, if we split the activities of the same person, Mila, in two sub sets, it is only for the sake of economic analysis. Actually, if Mila works, it is in great part to relax her budget constraint and increase the set of baskets she can afford to purchase. There is, indeed, a great deal of coherency in her activities.
• One could start therefore the analysis of Mila-producer saying that, like Mila-consumer, she wishes to satisfy her needs, or, it is the same thing, to maximize her utility. But because her activities qua producer are developed in a specific context, economists have proposed another starting point. They propose to analyse those activity not as utility maximizing, but as profit-maximizing.

Why should the producer maximize profit?

• Many objections have been raised against that assumption. Some say that the producer does not maximize profit because she has no idea of what that means. Indeed, as will be explained below, the maximization of profit requires a lot of knowledge and computational abilities which most producers do not possess. To that objection we can answer, however, that the same can be said of the consumer. True, the consumer does not compute marginal utilities, but chances are, if he is rational, that he will end up purchasing a basket that has more or less the property presented in the previous chapter (ratio of marginal utilities = ratio of prices). So if you accept that first objection you must reject as well the theory of consumer choice, and may be the principle of rationality. 2
• Another objection often raised is that the producer is interested by many things beside profit. For instance, she can care about her reputation and power and it is good for the reputation to be known as the owner and/or manager of a large company. In other words, the producer could care more about the annual turn-over, the volume of sales, than the annual benefits. And it is true that some business man enjoy a high reputation among layman even though their company is making losses. In the same spirit, it is often pointed out that many managers care more about their own comfort than about the profit realized. As long as they enjoy the large office, the services of the company car, and of course a high salary, they don't care that much about the profit realized.
• Still, another line of objection to the profit-maximizing hypothesis stresses the humanity of the producer. A producer, they say, often cares about the employees and will be ready to sacrifice part of the profit to avoid having to discharge an employee. She can also care for the consumer and leave her a greater part of the mutual gain to exchange than profit maximization would require.
• All the above objections contain some truth. It would be indeed stupid to argue that a producer never cares about reputation, power, the well-being of employees, or the happiness of the clients. But we have again three remarks to make that in part constitute counter-objections. First, some of the behaviour just describes are counterbalanced by other behaviour. For instance, if it is true that a manager of a large company might care more about his own well-being than about the profit distributed to the owners of the company, the owners will in general set up mechanisms to limit those deviances. We will come back to it when we will be discussing the firm in a later chapter. Second, if the producer wishes to reach those other goals, it will be very useful to make profits, and as much profit as possible! Indeed, can you maintain for a long time a large group of employees if you don't make profit? Can you serve the client properly if you don't invest, and can you invest if you make no profit? Also, if your office is “too big”, the company cars “too large”, and so on, you will have to transfer those extra expenses on the price of the good you produce, which means that you will sell less, and you will be in trouble. Finally, a related argument is that, even if it is true that some companies don't attempt to maximize profit, it is very likely that though the competition process, only those companies which realize profits will survive.
• For all those reason, we will hold on below to the hypothesis that the producer will act in order to maximize profit, profit being defined as the difference between revenues and costs.

Our goal: the determinants of the supply curve

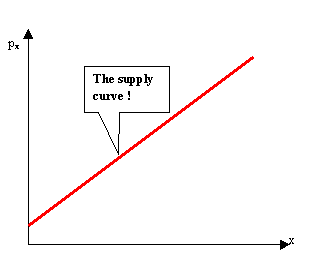
• If you think about it, the shape of that supply curve is not very intuitive, or at least, it is less intuitive than was the shape of the demand curve. Indeed, it was easy to understand that the consumer will buy smaller quantity of good x when the price of x increases, but why should the producer produce less of good x if the price decreases? Should he not always sell as much as possible? If you sell sandwiches will you say: well I will wait for the price of sandwiches to increase before producing more? May be yes, may be not. We will show below that the shape of that curve is in fact determined by, of course, the desire to maximize profit, but also the nature of the technology used for the production of good x .
• To arrive at the supply curve, we must therefore analyze the producer's choice. We already have decided to assume that this choice aims at maximizing profit. But the producer will have to face many constraints. Here is the list of those constraints:

- The technologies available for the production of that good

- The constraints imposed by the labour market

- The constraints imposed by the capital market

- The constraints coming from competition on the product market

- The constraints coming from consumer preferences

• The size of the profits will depend on how well the producer can deal with those constraints. The main one is to satisfy the needs of consumers, and this will be done by offering an interesting price/quality ratio. 3 But this in turn will depend of the choice of technology, and of the price and quality at which the producer can obtain the necessary “factors of production”: labour and capital. Finally, his pricing strategy will have to take into account the strategies of his competitors.
• As always, it is necessary to insist on the fact that the producer's choice will depend on the constraints as he perceives them at the time of his choice .

The technological constraint

• The technology permits the transformation of factors of production—also called inputs —into a product, also called output . There are many things that enter a production process: knowledge, time, human labour, machines, intermediary goods (like energy), etc. At that level of analysis it is usual to consider only two inputs: labour and capital. Furthermore we consider labour and capital to be homogeneous. In other words, we consider that there is only one type of labour and one type of capital. This is of course not true in reality. But, as always when creating a model of real life, our goal presently is to single out the essential, not to be as real as possible.
• Following those assumptions, a technology is a process by which those two inputs are transformed to produce one good. If we denote by L the quantity of labour entering the process, by K the quantity of capital and by Y the quantity produced, then a technology can be viewed as a function, noted F, associating to each bundle ( L , K ) a certain amount of production.
• 
• To be more precise, the function F gives the maximum output you can get when you employ a quantity K of capital and L of labour, and the corresponding technology. In real life, a firm does not always make the most efficient use of available inputs. For instance, employees may lack motivation, and the machines are rarely used at 100% of their possibilities. But we will assume for the moment that our producer does not have to face such problems.

Short run and Long run

• How much freedom has the producer in the choice of the production process is very variable. In principle he can choose the technology, and, given a technology, the combination of capital and labour that best serves his goal. But here again the economist likes to simplify thing, at least at the first stage of analysis. The simplification consists in assuming that the technology and the quantity of capital are given to the producer. They may for instance result from choices that have been made in the past. So that, for now, the only degree of flexibility left to the producer is the choice of the quantity of labour he wishes to employ.
• If you recall the form of the function of production, Y = F(K, L ), it is clear that when the function F is given and the stock of capital is fixed, the choice of the quantity of labour L is enough to determine the total output, Y .
• Now, to introduce some terminology, we will say that in that situation, the capital is a fixed factor of production and labour the only variable open to choice. And when the producer finds itself in such a situation we say that he is deciding for the short run . In the long run he will have the possibility to modify everything, including capital and technology. We will study decisions that have a long run effect in the next chapter. As indicated by the title of the present chapter, we will be here focusing on short run decision.
• You can also see the long-run, short –run distinction as something that specifies the time horizon of the decision-maker, here the producer. Let me explain what is meant by time horizon through an example. When, in a super-market, you decide what to buy for tonight's diner your time horizon is a short one: you think about what you had for lunch, and may be the other previous meals and you try to imagine how muck you would enjoy this or that tonight. May be you also think about what you must do the next day: pasta will be good if you go skiing, or running. But what you do next month is irrelevant to the decision. We say that your time horizon is short. Now, when you decide after high school whether or not you should go to college; or when you decide to buy an apartment or a house, you try (or at least it is a good advice) to picture the long term consequences of your choice. The time horizon is enlarged. In this chapter we study the behaviour of the producer when his time horizon is reduced.

The law of decreasing marginal productivity

• As was just said, in the short run, the quantity produced will entirely depend on the quantity of labour used. Therefore, for a given technology and a given level of capital, the possibility of production can be plugged on a curve that associates to each level of labour the level of output that can be obtained.
• The shape of that curve will of course depend on the technology we are considering and of how much capital we have. But there are good reason to believe that it will look like that:

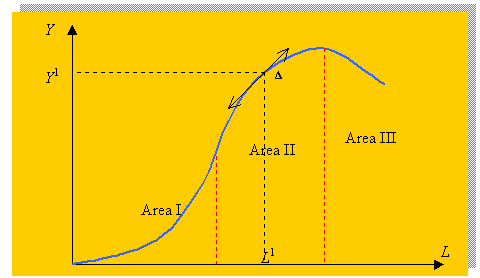
The short-run production function

• To comment on the shape of that curve it is useful to introduce some new concepts. . The first one is the concept of product . If with a quantity L of labour one can produce a quantity Y = F(K, L ), we say that Y is the product of that quantity L of labour. The second one is the concept of average product. The average product tells us how much product I can get, in average, with one unit of labour. Mathematically it is given by the ratio Y / L . If the producer chooses to use a quantity L 1 of labour, the average product is Y 1 / L 1 . 4 The second one is the concept of marginal product . We have already run into “marginal” quantities. Recall that the marginal utility of a good was that supplement of utility that I gain by consuming one more unit of that good. In the same fashion, the marginal product of labour is the supplement of production I can get if I use one more unit of production. Mathematically it is given by the tangent to the curve at the point considered. For instance, the marginal product of labour if I already employ a quantity L 1 of labour is given by the slope of the production function in A. 5
• Clearly, the average product and the marginal product are not constant; as we increase the quantity of labour they change. The graph has been divided in three areas. In Area I the marginal product of labour is increasing. The slope of the curve is becoming steeper and steeper. In Area II the marginal product is decreasing, but still positive. Each extra unit of labour allows an increase in production, but that increase is getting smaller and smaller. The last Area, Area III, is an area of negative marginal product: each extra unit of labour reduces the total quantity of product. The fact that marginal product don't keep increasing and becomes decreasing at some point is known as the law of diminishing marginal productivity .
• Now, clearly, the producer should make sure he never enters Area III! But in which of the first two areas should he choose to be? Before answering that question, let us stop a minute to explain why we usually have that succession of increasing, then decreasing, and finally negative marginal product.

The sources of increasing and decreasing marginal productivity

• We have all made that experience when starting a new task, like preparing for an exam, or writing a letter, or painting a room: the first minutes are not very productive, we need to organize ourselves. Then, once everything is organized, work starts to be efficient; our marginal product increases. But after a few hours (how many hours will depend of the person!), we start to loose our concentration, sometimes we get bored. Our marginal product starts to decrease. At some point, it is even preferable to stop working, like that student who has been working on the exam so many hours, and is so tired, that reading more bring confusion more than extra knowledge. We have entered Area III, the area of negative marginal product.
• Why do we go through those stages? Essentially because we have limited capacities . To make the parallel with the production function we say that the marginal product of labour start decreasing at some point because we have assumed a fixed amount of capital . As we enter Area II, and a fortiori in Area III, that shortage of capital is felt more and more by the producer.
• The name of the English economist, David Ricardo , is often associated to that observation. David Ricardo was living in the first part of the 19 th century, at a time when farming was still a large chunk of economic activities. For that reason Ricardo saw in the fact that land was limited (especially since he was living on an island!) an important source of decreasing marginal product, not only in farming, but in the national economy in general. But Ricardo is not the only one who has stressed the existence of decreasing marginal productivity and developed a certain form of pessimism. Today, the literature on sustainable development is a come back of those ideas. A full discussion of this literature will be presented later in the book. But at that point we can nonetheless say that we don't have to share that pessimism. Indeed, you don't need to have an angelic vision to understand that the scarcity of material resources can be counterbalanced by the creativity of human beings.
• What about increasing marginal product? Its sources are well known and were presented in part in chapter 3 (The dynamics of interaction). Division of labour is the main one. Just think about a new company; at the beginning one or two individuals are taking care of all the necessary tasks: production, marketing, accounting, public relations, advertising, relations with the public administration, etc. Then, as the company is hiring new employees, those tasks will be divided among all the employees according to each one competency. This greatly enhances productivity.
• If “more labour” is understood as some employees working more, then another phenomenon can increase marginal productivity: learning by doing . Indeed, as we repeat the same task, we discover new, more efficient ways of doing things. 6
• All this gives us an idea of the parameters that can shape that production function. More will be said later on that topic. After all, we are now dealing only with short run decision. In the long run, many other things can happen.

Introducing the costs of the factors of production

• Variable and fixed costs . Because some factors of production are given in the short run, whatever quantity the producer will decide to produce he will have to pay for those factors. For a company, it can be for instance the rent of the factory building, or the office, or the debt contracted in the past to buy the production tools. We will denote those fixed costs by C F . Again, in our story, this is the cost of capital. 7 No decision about it can be made in the short run. It is a constraint; an exogenous parameter as we like to say. On the contrary part of the production costs will vary with the level of production. That part forms what is called variable costs and will be represented by the function denoted CV( Y ). It is a function of the quantity produced, Y ; but since here the quantity produce depends entirely of the quantity of labour used, it can also be seen as a function of L .
• The total cost of the production of Y units of good, C( Y ), can therefore be written:

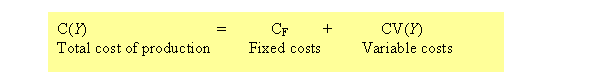
Assuming the cost of one unit of labour to be constant, then, if the production function has the shape presented in the previous graph , then the total cost function will look like that:

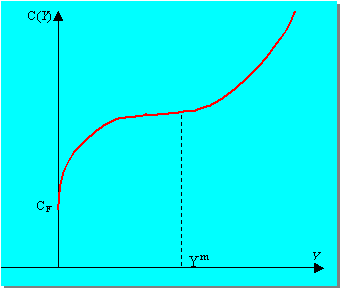
Total cost curve

• As we can see, even if nothing is produced ( Y = 0), the producer will have to pay C F . Then, as production is increased, total cost starts of course to rise. But it does so in an uneven way. Between zero production and a production of Y m , total cost first rises rapidly, and then continue to rise but less and less rapidly. More precisely, to produce the first unit the producer has to put more extra money than to produce the second one, and so on until we reach Y m . If we call the extra expenses that must be engaged in order to produce one more unit the marginal cost of production , then, what we observe is therefore that as production increases from zero to Y m , the marginal cost of production is decreasing. The reason for that is of course to be found in the technology. The fall in marginal costs is entirely due to the fact that marginal productivity tends to increase in Area I (see above). Follows a second area, from Y m on, where the marginal cost of production is increasing. If production is pushed a lot, marginal cost can become infinite. This is the point where it is physically impossible to increase production. This corresponds to the Area II and III in the previous gaph.
• It is frequent, instead of plugging the total cost curve on the graph, to plug directly the marginal cost curve, that is the curve that gives for each level of production what will be the extra cost necessary to produce one more unit. Not surprisingly, that curve is U-shaped. It reaches its minimum at Y m and then start to rise. 8

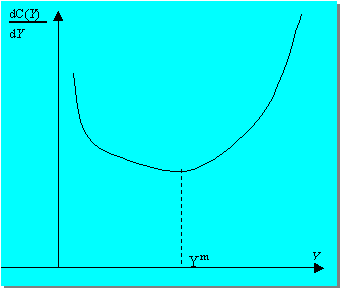
The marginal cost curve

The “market power” of the producer

• We are not far now from being able to identify the level of production that will be chosen by the producer. We know, by assumption, that he is trying to maximize profit and that profit is defined as benefits minus costs. We just studied the costs-side of the story; a few words about benefits are now in order.
• Benefits come from sales, sales depend on price, and how much consumers are ready to pay for that product depends on various parameters including prices of other goods, degree of substitutability between goods, and income. 9
• If our producer is the only one to offer a good that many wealthy consumers consider as irreplaceable and necessary, then he is a lucky man! He enjoys a great power on the market for that good. In particular he can set up the price he wishes without having anything to fear from competition, at least not in the short run. This case will be studied later when we will discuss monopoly.
• For now we will be studying what is almost the opposite case. A case in which our producer has very little power. In real life, we come close to that case whenever the producer is only one among many producers of that same good. As a result he is “forced” to set the same price as his competitors. The price is somehow “given” to him. He is a “price-taker”. But another consequence of our producer representing only a very small part of the total market supply is that he does not have to worry about sells; he can sell as much as he wishes at the “market price”.
• Hence, for now the level of benefits will be simply obtained by multiplying the quantity produced Y by the market price for that good that we will simply denote p .

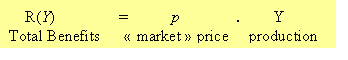
The choice of the producer

Profit, we said, is defined as benefits minus costs and will of course varies with the level of production:

P ( Y ) = R( Y ) - C( Y )

Profits Benefits Costs

Let us see graphically where that could be. In order to do so we just have to plug the benefits curve and the costs curve on the same graph and see where the distance between the two curves is the largest. (The benefit curve is easy to draw. It is a straight line going through the origin and with slope p ).

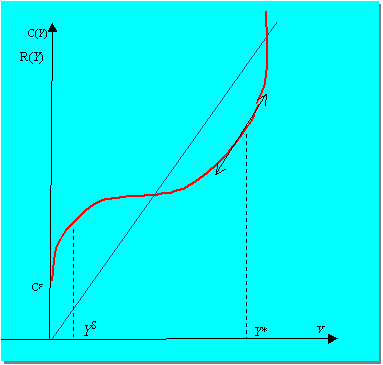
On that graph, the level of production for which the distance between the cost curve (the red curve) and the benefits curve (the blue one) is the greatest is Y S . But is it a good idea to produce a quantity Y S ? Surely not, because at that point the cost curve is above the benefit curve. So that point is in fact the point that maximizes losses! To find the level that maximizes profit we have to look at that part of the curve where costs are below benefits. We then find Y * to be the best choice.

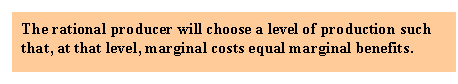
• Let us note that in our simple story, the value of marginal benefits is constant. Regardless of how much has already been produced, we have assumed that the producer can sell any extra unit at the market price of p . So marginal benefits is here always equal to p . This is why the benefits curve is a straight line. Later we will meet cases in which, if the producer wishes to sell more he will have to reduce the price; so that marginal benefits will no longer be constant.
• If you know some mathematics you probably have found the solution very rapidly. Indeed what we are looking for is the value of Y that maximizes profit, that is that maximize the function P ( Y ) = R( Y ) – C( Y ). This is a very simple function of one variable and we know that a necessary condition to get the optimum is that the first derivative be equal to 0. Since d P ( Y )/d Y = dR( Y )/d Y – dC( Y )/d Y , we deduce immediately that d P ( Y )/d Y = 0 if and only if dR( Y )/d Y = dC( Y )/d Y ; that is to say, if and only if marginal benefit equals marginal cost. 10
• Even though it is probably less intuitive, there is a second, but equivalent, way to arrive at that result. For that we have to start directly with marginal benefits and marginal costs. This is what we do next.

The choice of the producer: another, equivalent, approach

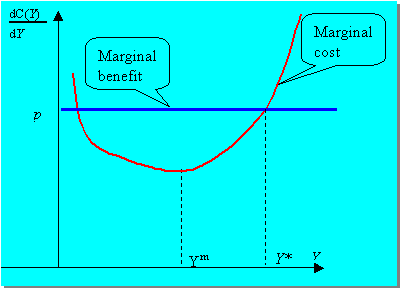
• We have already drawn the marginal cost curve. We just have now to draw the marginal benefits curve. As explained in the previous paragraph, in our simplify story the marginal benefits derived from the production of one extra unit is constant and equal to one. Once the new unit produced, the producer just has to go out and sell it at the market price of p . Hence if we superimpose the two curves we obtain the following graph:

• Let us note that, contrarily to what your intuition was may be suggesting you, the maximum of profit is not reached when the marginal cost is at its lowest (at Y m ). Even after that point it is profitable to produce, as long as the extra unit does not cost more to produce than it yields in extra benefit. Now, obviously, to go beyond Y * would be a mistake, because the next unit cost more than it yields.

The supply curve

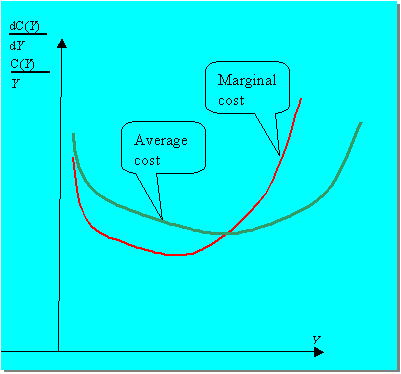
• The curve we need to draw is the average cost curve . (So far we have drawn only the total cost curve and the marginal cost curve). Now if you go back to what we said about costs, and if you look at the total cost curve, you should be able to deduce that the average cost curve, like the marginal cost curve, is U-shaped. And again, the reason behind that is to be found in the shape of the production function and the fact that the producer has a fixed amount of capital (recall the law of diminishing marginal product).
• Why do we need that curve? For a simple reason: it will remind us that the producer will not produce if he cannot do better than zero profit! Profit will be zero or negative whenever the benefits are below the costs, or, alternatively, whenever average cost (what it costs in average to produce one unit) is below average benefit (how much money I make, in average, from the sell of one unit). Average benefits here is simply again the price, p . And average benefit is given by the average benefit curve. 11

To draw the supply curve, let us imagine different market prices, p 0 , p 1 , p 2 , p 3 and see for each price where the optimal choice of the producer lies.

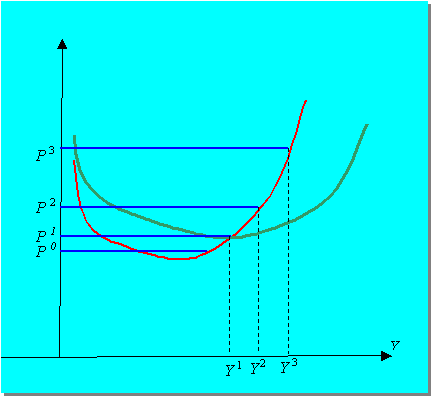
If the price is p 1 then the producer maximizes his profit setting at that point where the marginal cost is equal to marginal benefit (i.e., equals p 1 ). This is done by producing the quantity Y 1 . But since at that point the price equals average cost, it follows that at price p 1 the best that the producer can do is to cover his costs of production which leaves him with zero profit. Hence p 1 or, if you prefer, Y 1 sets a threshold below which it is not worthwhile to produce. This is known as the break-even point . If the price is below p 1 , if it is for instance p 0 , then the producer should close the shop! He will not have enough benefits to cover all expenses.

If the price is above p 1 , then the choice of the producer is given by that quantity for which the marginal cost is equal to the given price. So, if the price is p 2 , the producer will offer Y 2 , if it is p 3 , the producer will offer Y 3 , and so on. Hence we see that the supply curve—what the producer wishes to supply at the market price—is given by the marginal cost curve. More precisely, it is that part of the marginal cost curve which is above the break even point. Below, the producer does not produce anything. So let us draw the supply curve of our producer:

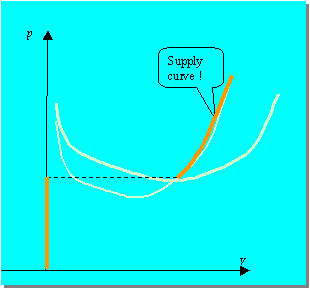
It has been a long chain of reasoning to reach that point! Remember that we started asking ourselves: Why does the supply curve slopes upwards? We now can answer that question. The short answer is that it looks like that because the marginal cost curve looks like that and because the desire to maximize profit leads the rational producer to produce until he reaches that point where price equals marginal cost. But an honest answer should also make clear that it will not necessarily look like that! We understand that the shape of the producer's supply curve depends on the shape of the producer's marginal cost curve, which itself depends on the production function, and in particular of the evolution of marginal productivity. True, the shape we have assumed for the production function and that led us to that specific supply curve is a very plausible one, especially in the short run when some factors of production are fixed. But this is an empirical matter, not a matter of logic.

Based on our analysis we can also address the question of the elasticity of the supply curve . We have already met the concept of elasticity in the chapter on consumer's choice. The idea is to measure the sensibility of a choice to a variation in one of the parameters of that choice. When economists talk about the elasticity of supply, they usually mean the price elasticity of supply. So, that elasticity helps us to answer questions such as: if there is a 10% price increase, in which sense and in what proportion will the quantity supplied change? The formula is of the usual type for an elasticity:

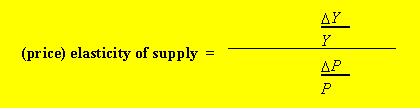
So, what can we say, based on our analysis, about that elasticity? Not too much unless we are given the exact form of the production function. 12 We have seen indeed, more than once, that the choice of the producer depends to a large extent of the shape of that function. Unless we know the shape we cannot say anything precise. Something we can say however is that if the price falls below the break-even point, then indeed the consequence in terms of production can be important.

From individual supply curve to the market supply curve

The market supply curve in the short run is just the summation of all the supplies coming from the various firms that produce that good. If all the individual supply curves are upward sloping then the curve that aggregates those individual curves will be increasing as well. That's almost all one can say about the market supply at that point.

1 Schizophrenia: Any of a group of psychotic disorders usually characterized by withdrawal from reality, illogical patterns of thinking, delusions, and hallucinations, and accompanied in varying degrees by other emotional, behavioural, or intellectual disturbances.

2 The Noble Prize winner, Herbert Simon, has argued that producers, instead of attempting to maximize profits attempt to reach a level of profit that appears “satisfactory” to them. This is probably a fair description of the producer's behaviour. From an analytical point of view, it raises however a new problem: how does the producer decide of what would be “satisfactory”? And when trying to answer that question, the shadow of profit maximization is reappearing.

3 See our discussion at the beginning of chapter when we explained why we start by studying consumption.

4 Graphically, it is given by the slope of the line going from the origin to the point considered. Hence the average product when you use a quantity L 1 of labour is the slope of the line going from the origin to the point A.

5 For the mathematically inclined, the marginal product of labour is defined at that variation of product D Y that I get when I use one more unit of labour. If one can consider arbitrarily small variations of labour, like working one more second, then the marginal product is defined as the ratio D Y/ D L. As D L goes to zero, this ratio tends toward the derivative of Y with respect to L, what is usually denoted dY/dL, or if you prefer, dF(K,L)/dL. This is by definition the slope of the tangent to the curve at that point.

6 Here we could insert a footnote on the findings of Arrow in his study of factories productivity.

7 In real life, if you talk to the manager of a company, he or she will probably tell you that a large part of labour costs are fixed in the short run! You cannot lay off someone easily, and often you don't want to. It is sometimes faster and easier to buy a new computer, or any new machine. But the fact that labour costs are sometimes “fixed” in the short run does not change the main conclusions our story will lead us to.

8 Mathematically, the marginal cost is computed by taking the first derivative of the total cost function, dC( Y )/dY . This makes sense because the derivative is precisely the limit of the ratio D C( Y )/ D Y when D Y becomes small, that is to say, it gives us how much more it will cost ( D C( Y )) to produce a little more ( D Y ).

9 See the chapters on consumer's choice.

10 If you know just a little more mathematics, you know that it is useful to check the sufficient conditions to make sure that you are at the maximum (and not at Y S ! ). And the second order condition says that at that point marginal benefits must be increasing less rapidly than marginal cost (or, that it must be decreasing more rapidly).

11 That curve was not drawn entirely at random. In particular the fact that it is cut by the marginal curve from below and precisely at that point where the average curve reaches its minimum is something that can be proven.

12 If we have a mathematical formula for the production function, then it is quite easy to give the formula for the elasticity. The maximisation of profit gives us the quantity produced as a function of the price p . We then just have to take the derivative of that quantity with respect to p , divide the result by Y and multiply by p . In other words we compute (d Y *( p ) / d p ) . ( p / Y *( p )).